Reading time : 10 minutes

I bought a roll of wrapping paper.
I know, Caroline… it’s not very environmentally-friendly.
But now that it’s done, now that I have this roll of paper, what can I do to make the best of the situation?

It’s true, Caroline, I could return it!
But before I do that, if it’s OK with you, I want to try to figure out how I can wrap as many presents as possible with this roll!
So, what are the dimensions of this roll?
To begin with, let’s agree that it’s not the roll itself but rather what’s wrapped around it that’s of interest!
The package only provides the surface area: 12m2
That’s interesting, but it won’t help me very much given that there are infinite length/width combinations of paper with an area of 12m2.
Here are 2 examples:
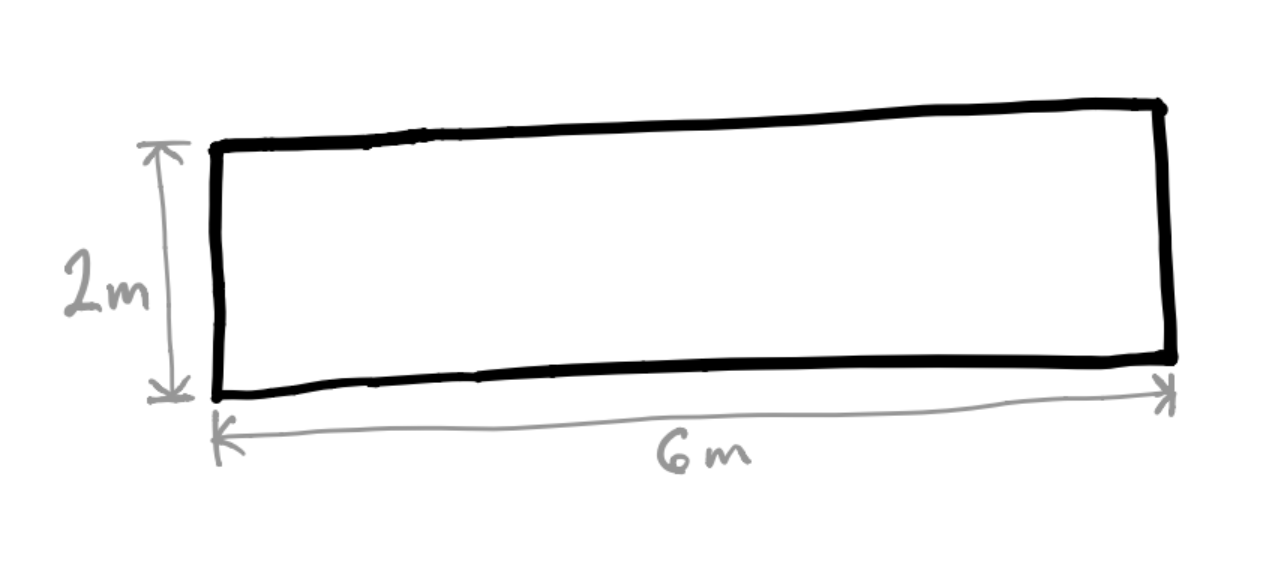
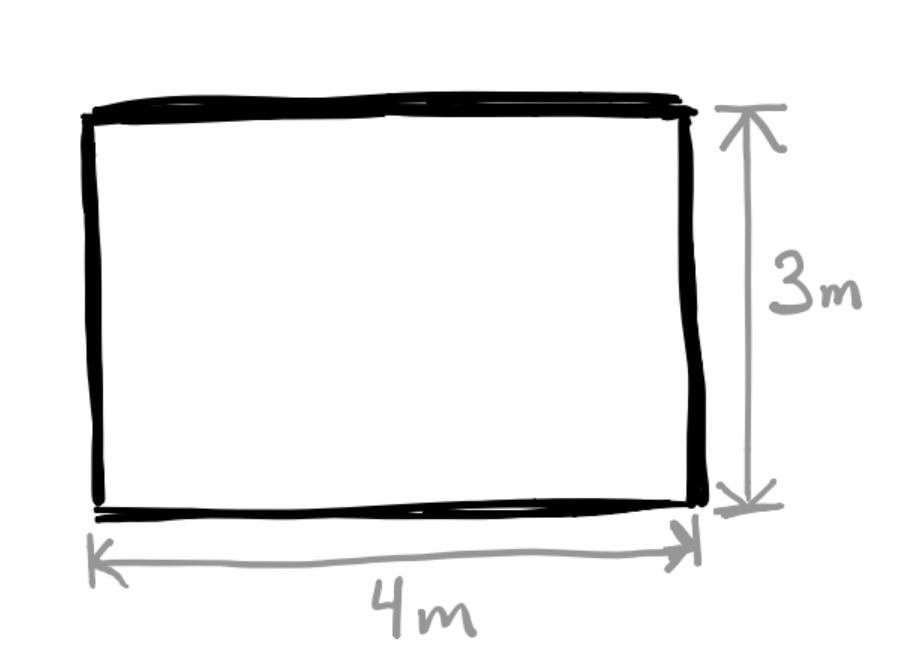
Give me a minute. I’m going to go measure. I’ll be right back.
…
…
Ok, I’m back!
The width of the roll is 100 cm, so the length is about 12m
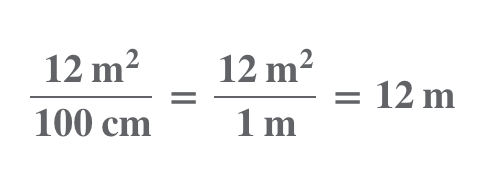
In total, I have a piece of paper that is 12m long and 100 cm wide.
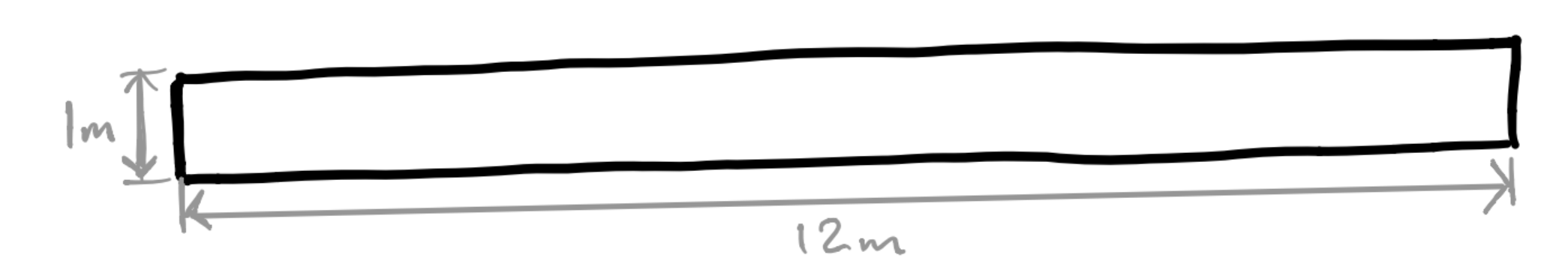
Good! Now that I know that, what can I do to use the paper as efficiently as possible to wrap the presents?
First, let’s say I have a cubic present to wrap. And to keep things simple, let’s say that the measurement of one side is 1 unit (u).
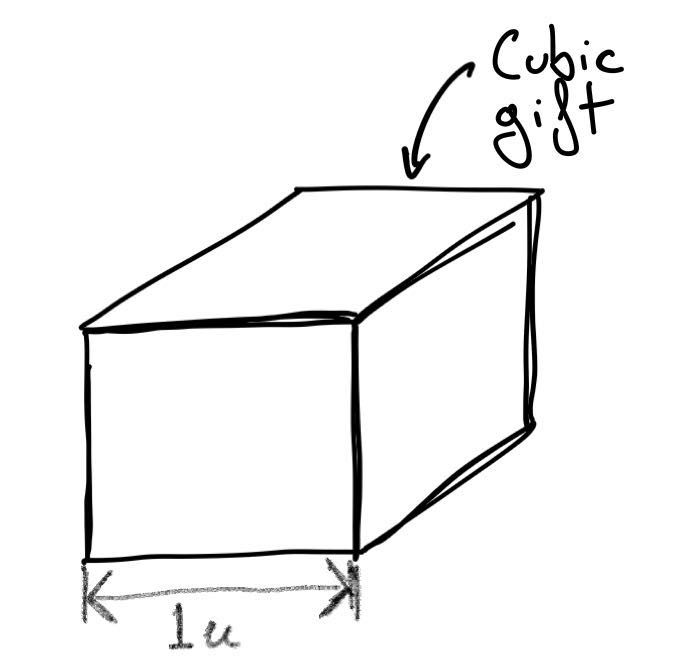
350
Normally, I like to keep things as simple as possible:
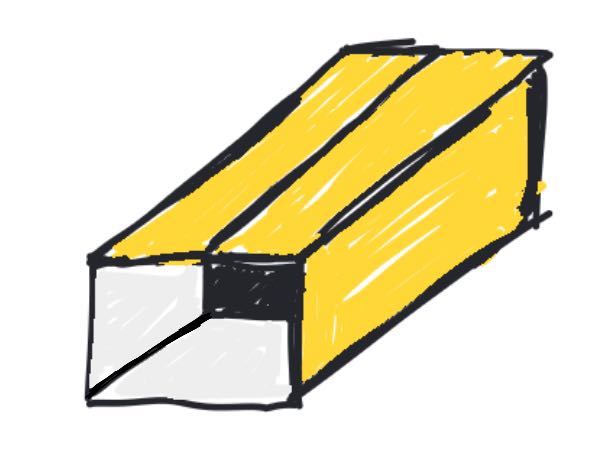
I try to bring the 2 sides of paper together so that they meet at the centre of the top surface.
Here’s a plan view of the present placed in the centre of the wrapping paper.
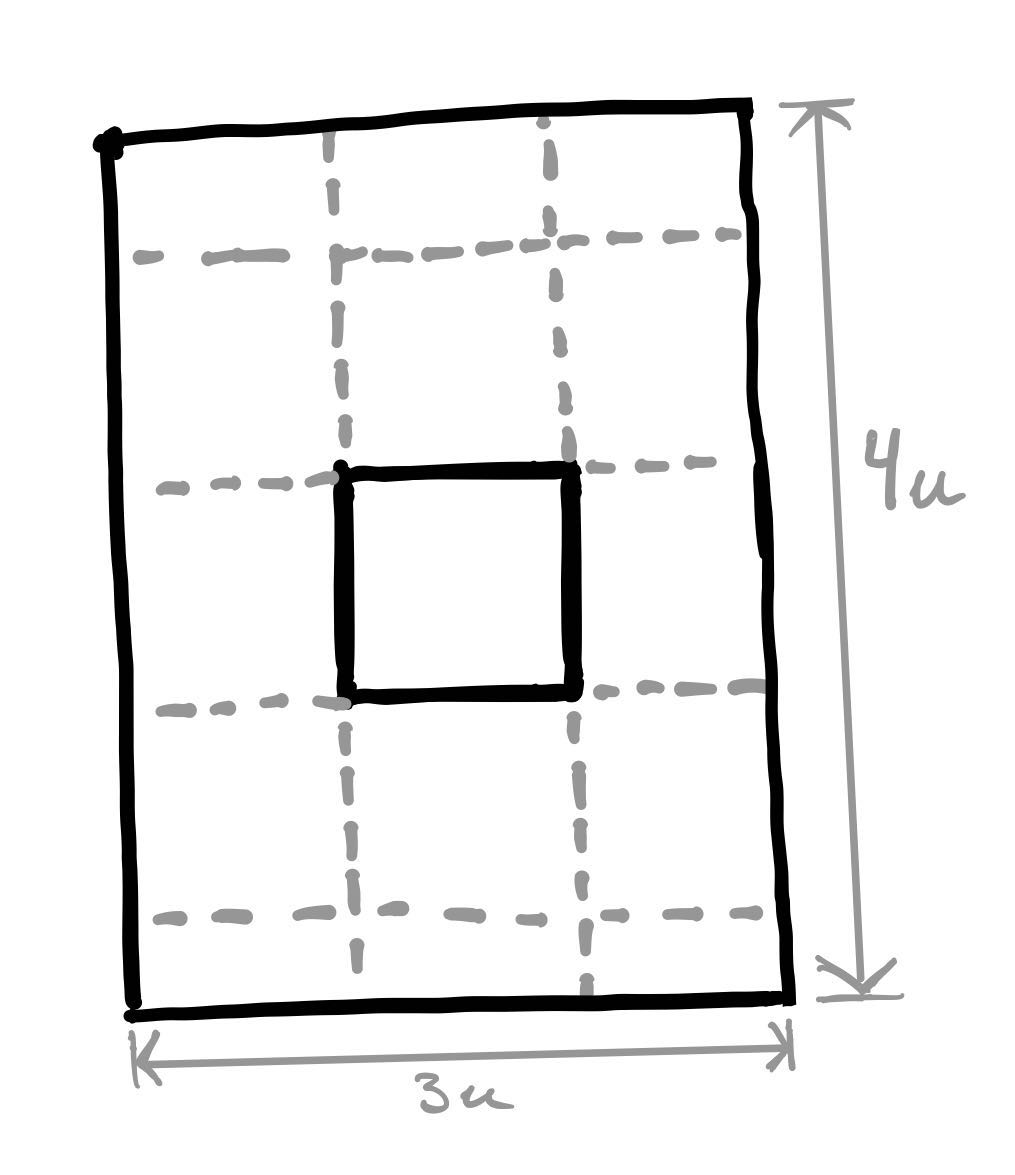
Vertically, I want the 2 sides of the paper to meet in the middle of the top of the box, which means I need about 4 times the length of the box.
Horizontally, I keep about 3 times the width of the box so that the flaps cover the sides. I know it’s not ideal, but I’m trying to reproduce the classic approach of a fairly experienced gift wrapper (me!).
To summarize, I used a surface area of 3×4 = 12𝐮2 to cover the present.
Of these 12𝐮2, the surface area of the cubic box represents 6𝐮2.
This means 6𝐮2 were wasted. This is the surface area of the paper that was not used to cover the cube.
No, Caroline, that’s not great at all.

So, what can I do to improve the situation? Wait! I think I have an idea!
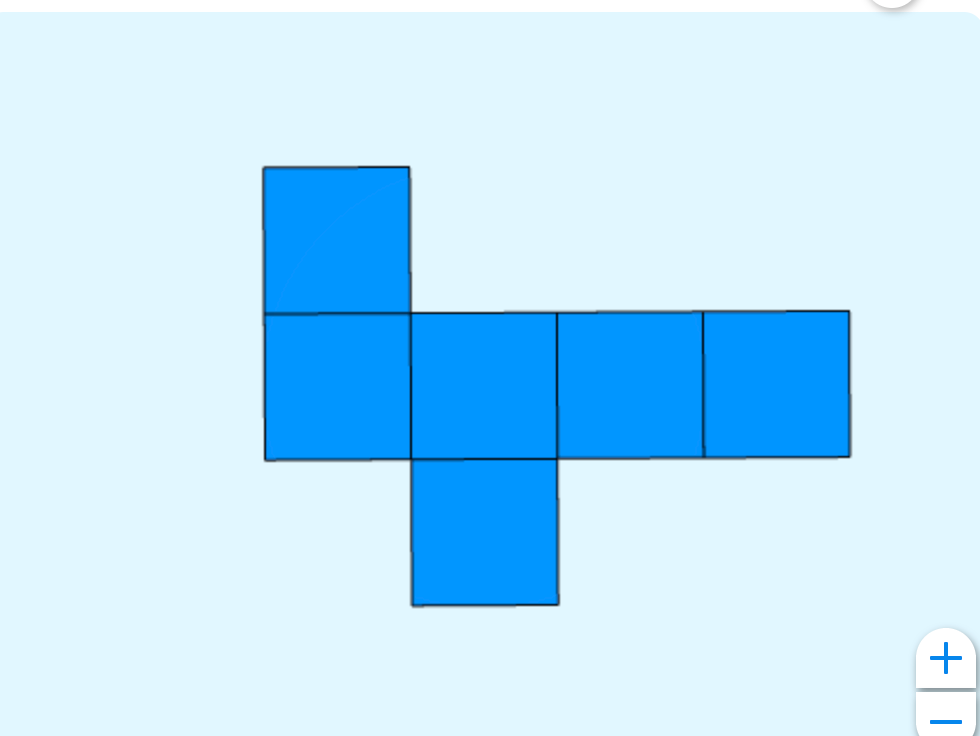
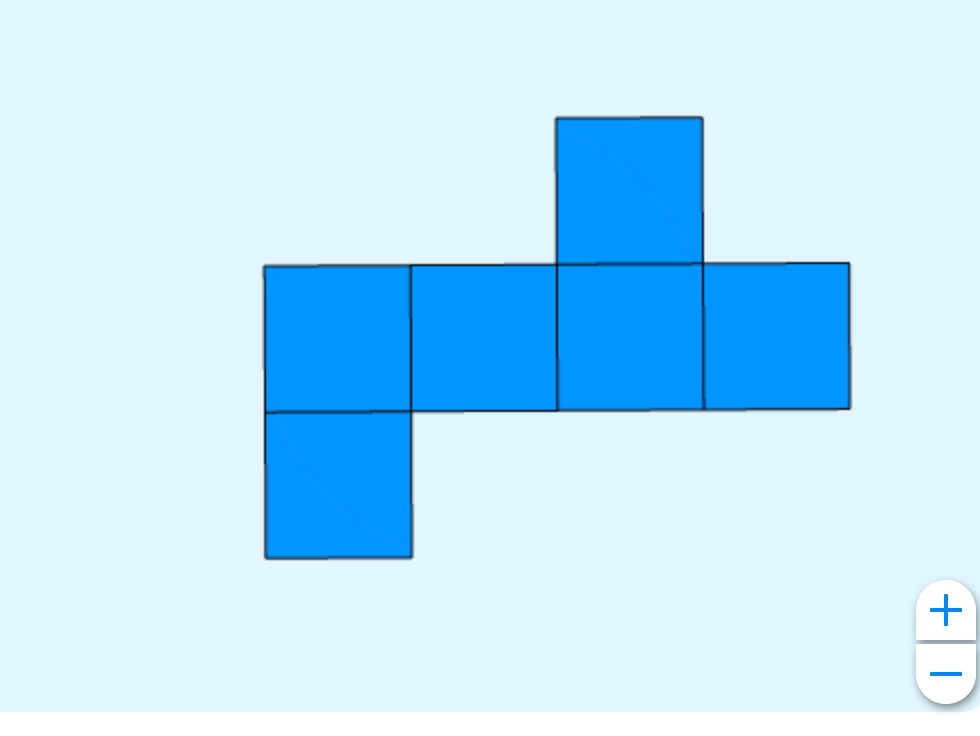
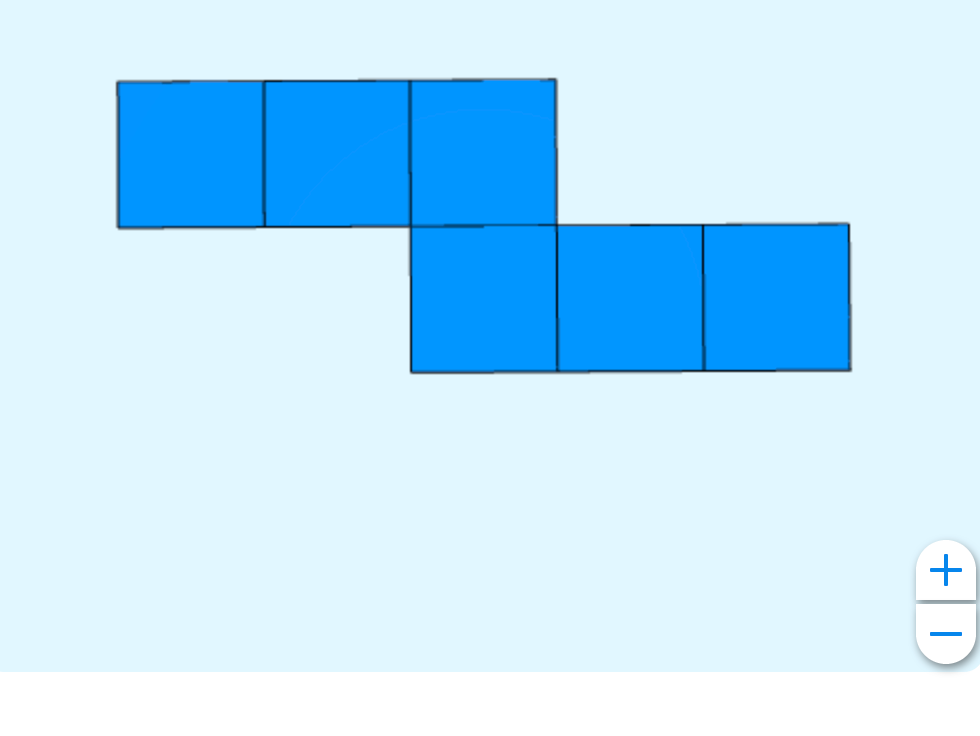
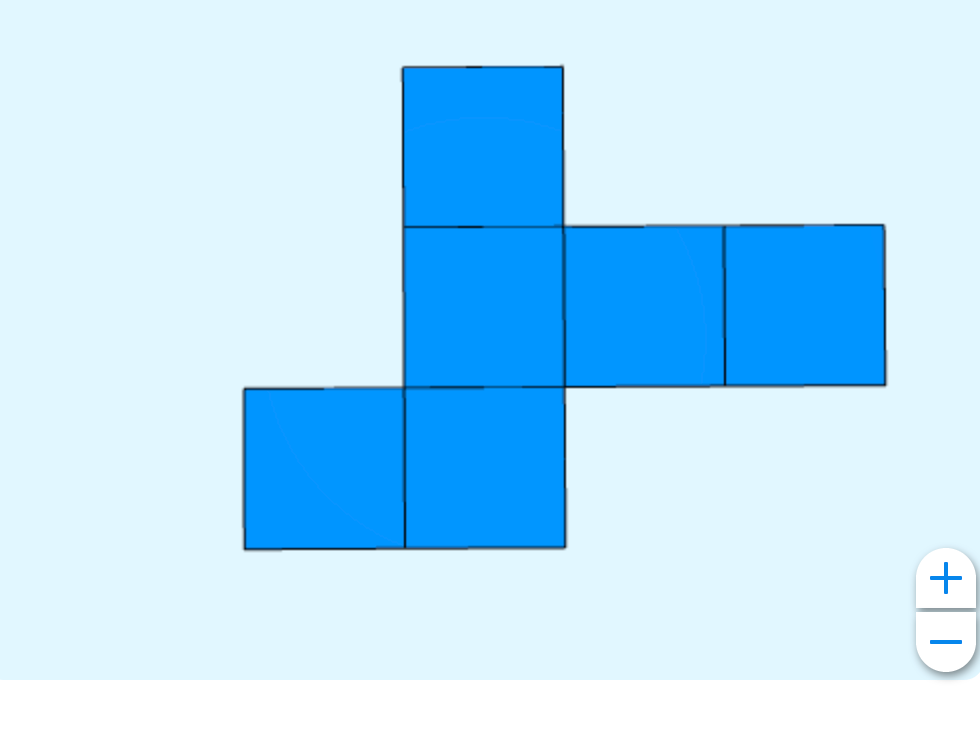
I’m going to try using the net of the cube.
Even better, I just realized I can use the tool from Netmath to represent it!
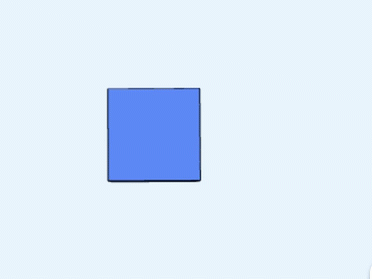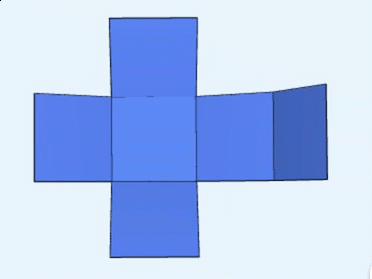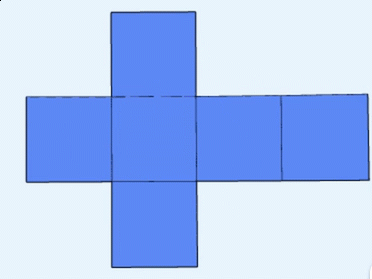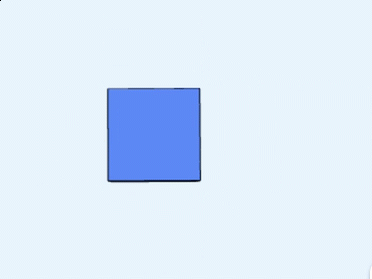
Note that the net I chose here is just one of several possibilities! In fact, how many nets does a cube have?
If I cut the wrapping paper to correspond exactly to the net of the cube, there will be 0 loss.
Z. E. R. O.
The ratio would be 1 : 1. Each square centimetre of paper would be used to cover a part of the present. That’s amazing!
But, it’s not very practical because…
- … I’ll need to use a lot of tape to attach all the sides
- … if I make a crooked cut, there will be a gap exposing part of the present
- … there will probably be lots of bits and pieces of unused paper
- … it’ll take too long
Nevertheless, let’s use this net:
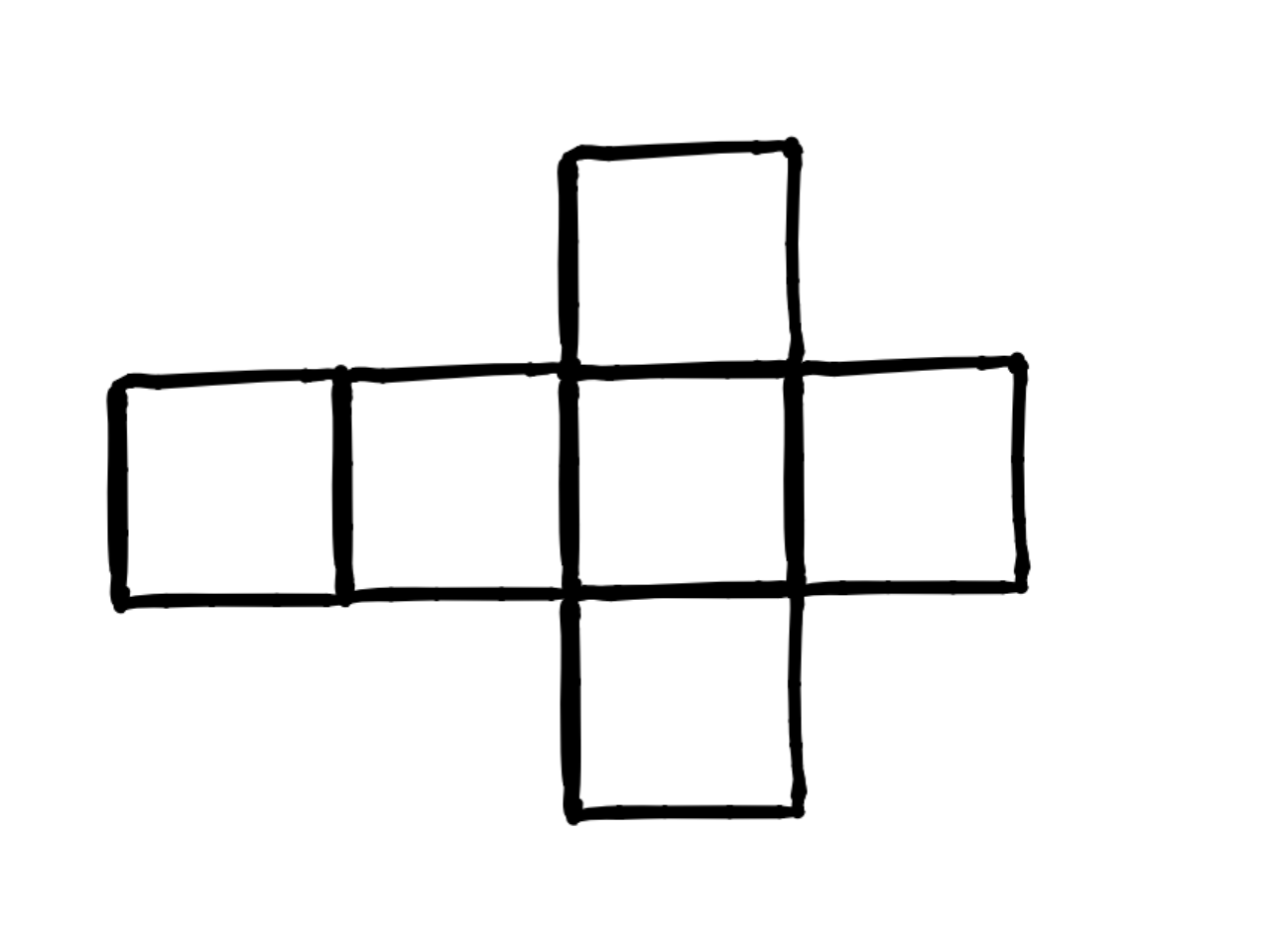
Here’s my idea!
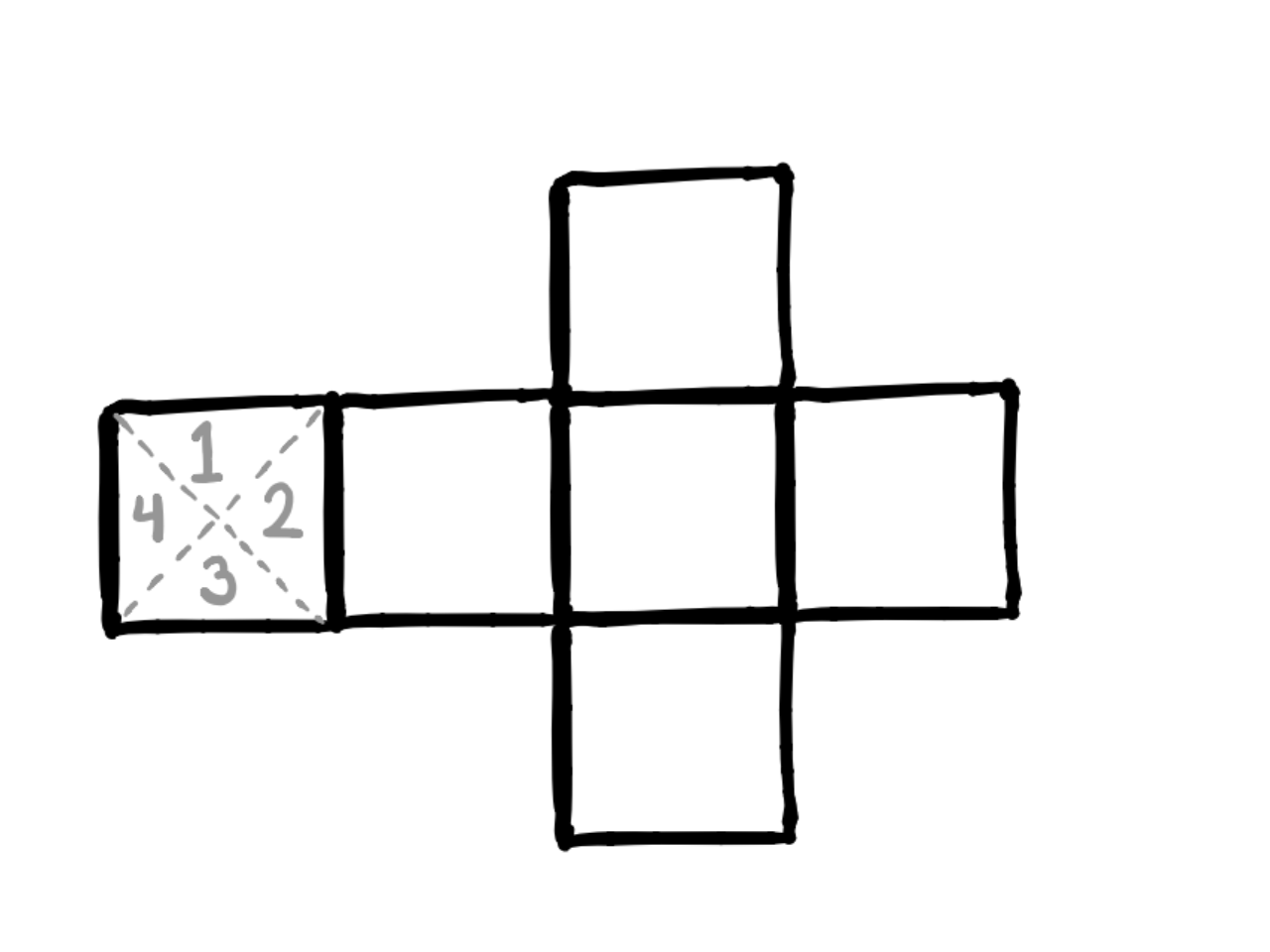
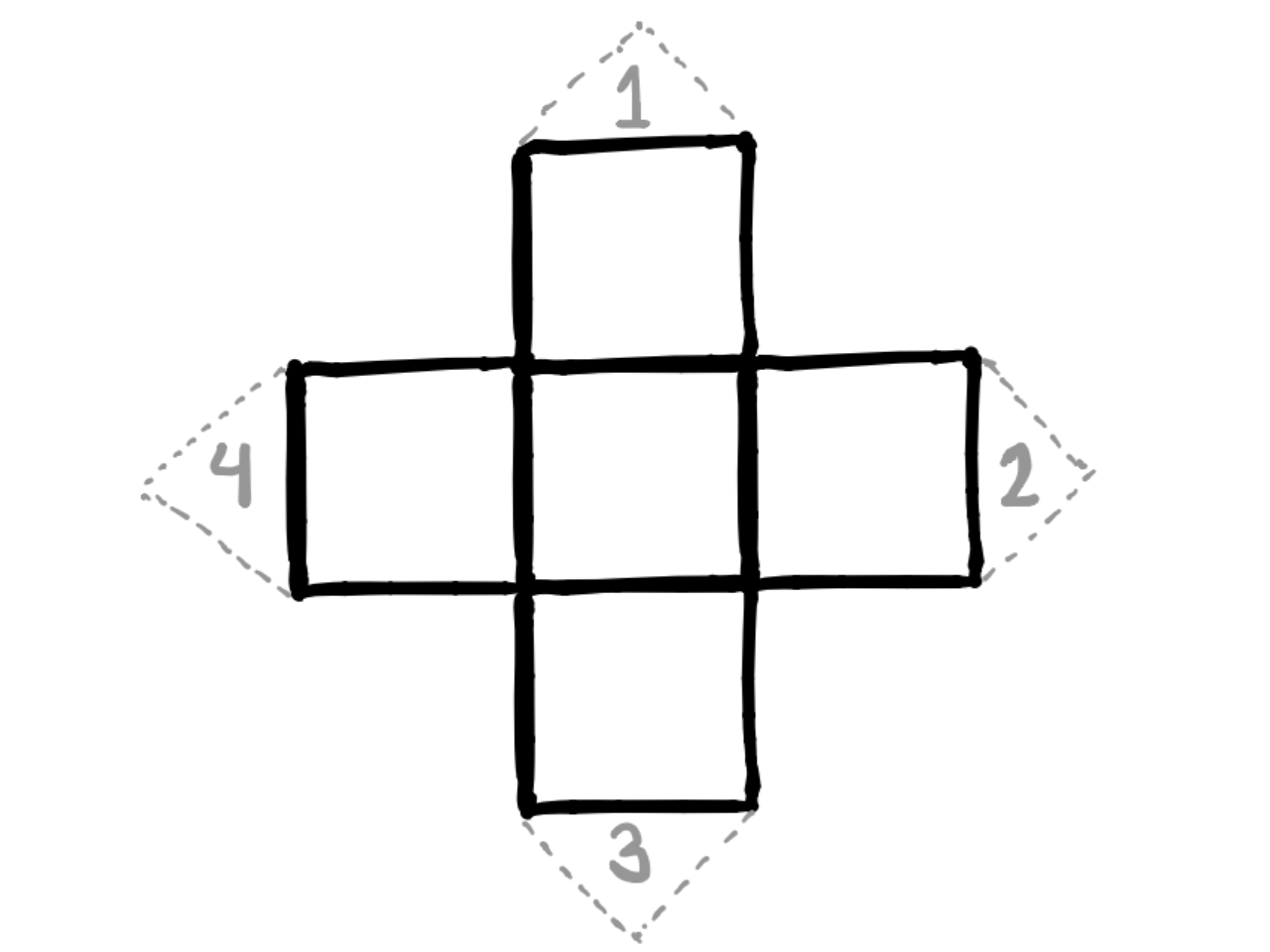
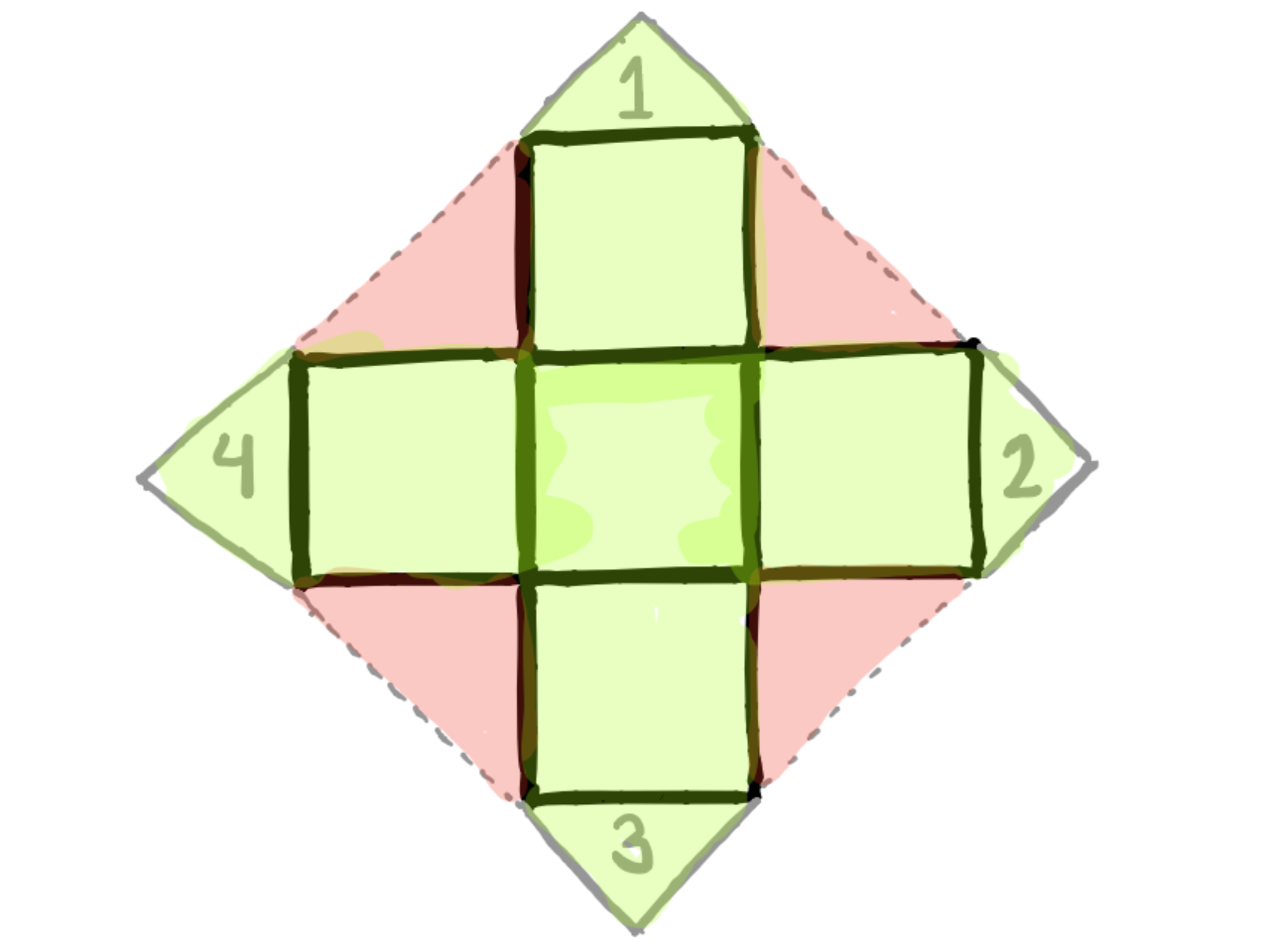
In comparison with the preceding image, if we consider that the central square (one side of the present) has a surface area of 1 square unit (u2), then we have a total surface area of with a loss of 8 u2 with a loss of 2 u2.
There are 2 interesting observations to be made here:
- Less paper is used by placing the cube diagonally.
- A greater proportion of the paper is used to cover the cube (present).
If you come up with an even more efficient method, please let me know! In the meantime, I’m off to return the roll of paper!
After all, it’s way more efficient to just use a pillow case!