Secondary 1 to 5
Reading time: 10 minutes
Netmath’s mission is to help every student discover and develop their own mathematical potential while having fun.
In this edition of “Simon is wondering…”, techno-pedagogue and Netmath expert Simon suggests that you tackle an everyday question from a mathematical perspective with your students. This approach will help them recognize that math can be really fun when it comes to better understanding the world around us.
Today, we are talking about sequences !
I’ve known what sequences are for some time now, but I recently had a crazy thought: Can I hear a sequence of numbers?
The first step in answering this question is to clearly define what a sequence is.
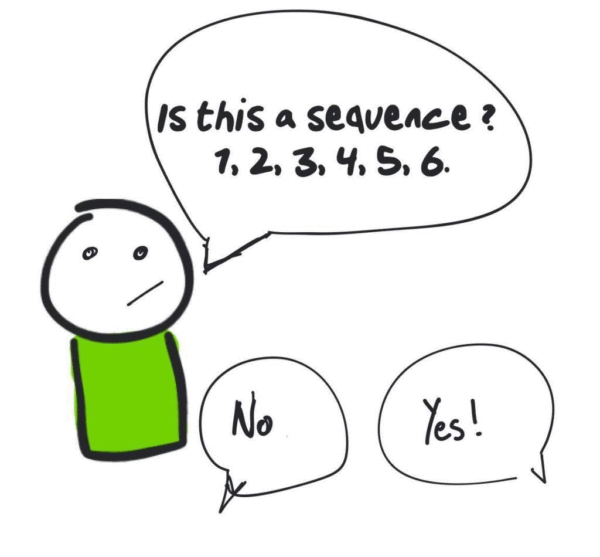
In fact, this is what’s called a finite sequence. It’s finite because it doesn’t go on for infinity.
OK! So, let’s see how I can represent the fact that the sequence can go on and on for as long as we want!
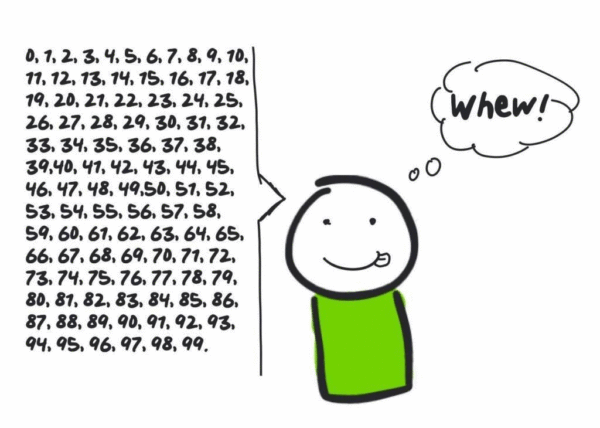
Whew! It’s not easy going on for infinity! There must be a way of indicating that we want to continue on and on and on, without having to write each number in the sequence! But how?
After doing a little research, I discovered that we use ellipsis points (…) to indicate that a sequence keeps going.

Well, if you take a closer look, you’ll know when to stop.
0, 1, …
How can you deduce the rest of the sequence? Here are a few possible sequences that start with 0 followed by 1 :
- 0, 1, 0, 1, 0, 1, 0, …
- 0, 1, 3, 7, 11, 16, …
- 0, 1, 2, 3, 4, 5, 6, …
But which one should you choose? Inversely, it takes much too long to write 100 terms before adding the ellipsis points.
Logically, we need to write down just enough terms to see the pattern (usually, 3 terms are enough). If you look at the 3 sequence in the example, the 3rd term is always different.
- 0, 1, 0, 1, 0, 1, 0, …
- 0, 1, 3, 7, 11, 16, …
- 0, 1, 2, 3, 4, 5, 6, …
So, by specifying the 3rd term, we can more precisely determine what the sequence is.
Let’s summarize: A sequence is written with numbers that are called terms, which are separated by a comma, and if a sequence is infinite, we add ellipsis points after having written down just enough terms to understand the pattern.
Now I think we’re ready to tackle the question head on! How can we hear a sequence? Let’s use the example of the sequence 0, 1, 2, …
If we associate a musical note with each number, we can listen to the numbers in any sequence! OK, so how do we do that?
Hmmm… I’ve got it! Google often has the answers to our questions. We just have to search a little! Here’s the Chrome Music Lab!
Let’s see what our sequence (1, 2, 3, …) looks like in this context. All you have to do is click HERE.
OK, it’s not exactly the most exciting musical sequence, but we can have a lot of fun with this tool!
I wonder how we could make this a little more melodic…
I know! Let’s try using known numbers and taking their decimal development as the sequence of numbers. For example, the beginning of the sequence with decimals of the number pi would be: (1, 4, 1, 5, 9, …).
Listen to what the first 100 decimals of pi sound like by clicking HERE.
And the first 100 decimals of e (the base of the natural logarithm) by clicking HERE.
And finally, the first 100 decimals of phi (the golden number).
Well, this is definitely becoming more interesting, but it’s difficult to distinguish the variations between the sequence because for each sequence, the order of the terms is practically random.
But when we listen to music, there’s a pattern, which is what gives a piece melody. Our brains get very excited when they detect a pattern!
So, I could try to choose decimal developments that are periodic. In other words, the same parts are repeated. This is what happens with rational numbers. Basically, a rational number is a number that can be expressed as a fraction. Let me be more precise:
This time around, I’m a little more convinced. Convinced that this can serve as the basis for a piece of music. While these sequence could never replace all the wonderful musical variety that musicians create, math can certainly inspire them! Check out this masterpiece by Wintergatan: https://youtu.be/IvUU8joBb1Q

